Three-Dimensional Symmetric Shift Radix Systems

About the paper

coauthors Andrea Huszti
Klaus Scheicher
Jörg M. Thuswaldner
language English
published in Acta Arithmetica 129, No. 2 (2007)
pages 147 to 166
DOI 10.4064/aa129-2-2
supported by FWF, project S9610 (NFN S9600)
Foundation "Aktion Österreich-Ungarn", project 63öu3

Abstract

Shift radix systems have been introduced by Akiyama et al. as a common generalisation of β-expansions and canonical number systems. In the present paper we study a variant of them, so-called symmetric shift radix systems which were introduced recently by Akiyama and Scheicher. In particular, for d∈N and r∈Rd let (a=(a1,…,ad))
τr: Zd → Zd, a→(a2,…,ad,−⌊a1r1+a2r2+…+adrd+½⌋).
The mapping τr is called a symmetric shift radix system, if
∀a∈Zd ∃k∈N: τrk(a) = 0.
Akiyama and Scheicher found out that the parameters r giving rise to a symmetric shift radix system in R2 form an isosceles triangle together with parts of its boundary. In the present paper we completely characterise all symmetric shift radix systems in the three dimensional space. The result is that r∈Rd gives rise to a symmetric shift radix system τr if and only if r is contained in the union of three convex polyhedra (together with some parts of their boundary). We describe this set explicitly.

References

  1. S. Akiyama, T. Borbély, H. Brunotte, A. Pethő, J. M. Thuswaldner, Generalized radix representations and dynamical systems I, Acta Math. Hungar. 108 (2005), 207—238.
  2. S. Akiyama, H. Brunotte, A. Pethő, J. M. Thuswaldner, Generalized radix representations and dynamical systems II, Acta Arith. 121 (2006), 21—61.
  3. S. Akiyama, H. Brunotte, A. Pethő, J. M. Thuswaldner, Generalized radix representations and dynamical systems III, Osaka J. Math. 45 (2008), 347—374.
  4. S. Akiyama, K. Scheicher, Symmetric shift radix systems and finite expansions, Math. Pannon. 18 (2007), 101—124.
  5. H. Brunotte, On trinomial bases of radix representations of algebraic integers, Acta Sci. Math. Acta Sci. Math. (Szeged) 67 (2001), 521—527.
  6. G. E. Collins, Quantifier Elimination for the Elementary Theory of Real Closed Fields by Cylindrical Algebraic Decomposition, Lect. Notes Comput. Sci. 33 (1975), 134—183.
  7. C. Frougny, B. Solomyak, Finite beta-expansions, Ergod. Th. and Dynam. Sys. 12 (1992), 713—723.
  8. W. Parry, On the β-expansions of real numbers, Acta Math. Acad. Sci. Hungar. 11 (1960), 401—416.
  9. A. Pethő, On a polynomial transformation and its application to the construction of a public key cryptosystem, Computational number theory (Debrecen, 1989), de Gruyter, Berlin, 1991, 31—43.
  10. A. Rényi, Representations for real numbers and their ergodic properties, Acta Math. Acad. Sci. Hungar. 8 (1957), 477—493.
  11. I. Schur, Über Potenzreihen, die im inneren des Einheitskreises beschränkt sind, J. reine angew. Math. 148 (1918), 122—145,
  12. P. Surer, Characterisation results for shift radix systems, Math. Pannon. 18 (2007), 265—297.
  13. P. Surer, Personal homepage, http://www.palovsky.com/links/p22007.htm.
  14. T. Takagi, Lectures in Algebra, 1965.

Animated figure

This animated figure shows the subset of the R3 whose points induce symmetric shift radix systems. The red parts of the boundary are contained in the set, while the green parts are not.

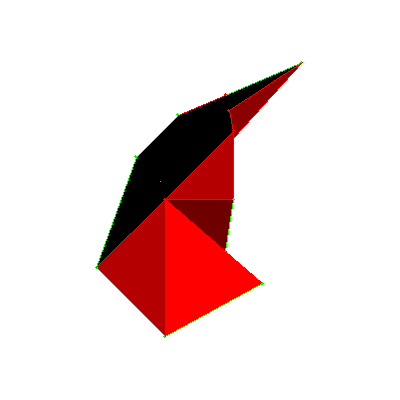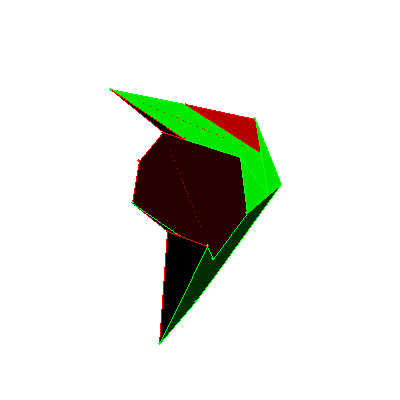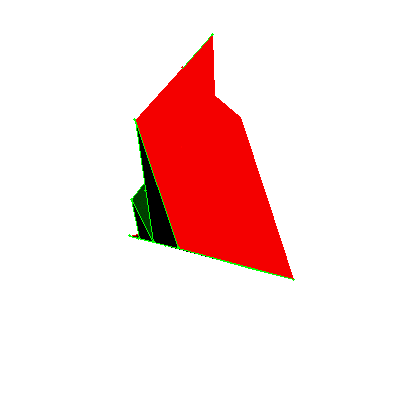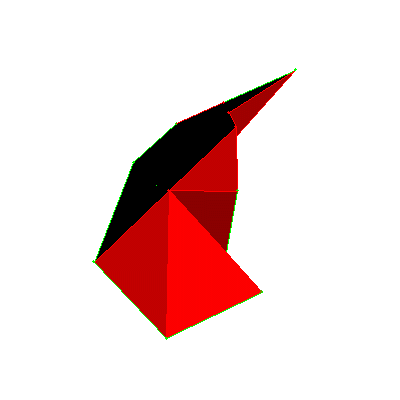
model of symmetric shift radix systems in the three dimensional space

Download

Mathematica® Notebook-File (Version 5.1) with an implementation of the algorithm FP.
download FP

Mathematica® Notebook-File (Version 5.1) with an implementation of the algorithm VC.
download VC

Links

Acta Arithmetica
Austrian Science Foundation (FWF)
National Research Network (NFN) S9600
Foundation "Aktion Österreich-Ungarn"